a symmetry break down
Backstory
A bit of backstory why i inadvertently chose adichotomy. Well, nothing really profound, but it was mostly do to with irony. Asymmetry and asynchronous came to mind and i just ran with it. We don’t tend to infer that there is a preference to things when we talk about reality. But, it seemed pretty obvious that we tend to prefer stuff like synchronicity, symmetry and duplicity (think lots of hundred dolla’ bills y’all). We just like simplicity and i think, as creators, we tend to create things we tend to prefer. Of course this isn’t written in stone. Other than the one rule i gave you in the previous post, i can’t seem to find another. Oh, well. I amuse easily sometimes.
I was going to use something about shit being typically asymmetrical, and why it somehow seems more pleasing if you manipulate it into a sphere, but i couldn’t figure out the humor there, irony can be an odd duck at times.
But we (therefore reality) tend to enjoy simplicity and for that, symmetry is where it’s at. Something about the earth (most massive bodies in space) generally could be assumed to be symmetrical by nature, until acted upon. The earth is symmetrical, but those pesky massive bodies (read: the moon, planets, the sun, etc) keeps pulling out of shape. Regardless, it can still be divided equally, fairly simply either way.
Math breaks down too
The sciences love math, they really prefer it over most other ways of deciding what the rules/laws of reality are. And why not, it’s pretty elegant. But it’s makes painting a complete picture really hard.
Don’t have a scientist try to work on your car.
While many scientists can explain to you how a combustion engine works. Modern complexity (without experience) of a combustion engine might leave them pretty baffled as to why it won’t operate. Don’t get me wrong, i’m sure given enough trial and error, i’m sure many can dive in and figure it out. But, yanno, we leave it to the experienced professions, it’s all about perspective knowledge (read: frame of reference).
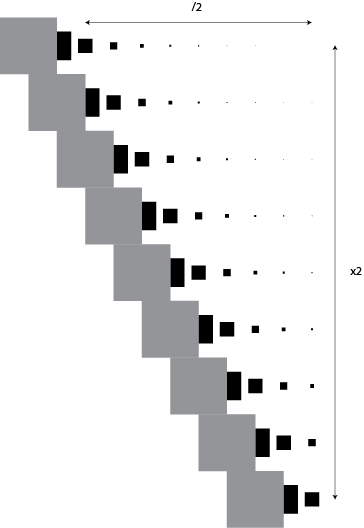
Anyway, right, math breaks down. Below are 2 illustrations, one (the first) can be considered a 2 dimensional explanation of how size breaks down, even mathematically. The second will attempt to explain in more of a 3d variation. Please note the rule prior “no perfect duplicity“.
Using 10 digit math 0-9, why math breaks down. I used squares because they like to fit together better than circles. But, like i said, it really seems like reality enjoys the infinite random calculations of pi. Go figure.

Same as above, only we go 3d (objects in front/behind). Again, remembering our one rule.
These are transparent images, so if you’re using reader view in dark mode, they probably won’t translate well.
As you can see, these are really simple illustrations about dividing/subtracting and multiplying/adding objects (or numbers too). But as you might be able to see, as they near 0 and 1, they lose the fight. They either end in infinite or finite (again remembering our rule). If you ask a mathematician, they would hopefully confirm this phenomenon. So, you really always have to be viewing the whole to get the complete picture and leave the bits to dissolve. Regardless, even seasoned mechanics use the trial and error method, they just happen to be better equipped to solve a malfunctioning engine.